

Next: An application
Up: Research interest / own
Previous: Cluster Operator Parameterization
Toggle Background
According to
equations (16), (17),
(19), (20)
and (21) we finally summerize and define our wavefunction as
and the cluster operator as
Inserting equation (22) into the Schrödinger equation
we get
Projecting equation (24) from the left
onto
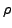 |
| 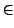
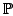
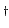
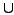
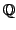
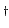 we obtain a
system of equations linear in the reference coefficients c
we obtain a
system of equations linear in the reference coefficients c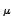 and
non linear in the amplitudes t| i
and
non linear in the amplitudes t| i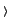
with the additional equation
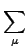 | c | c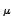 |2 = 1 |2 = 1 |
(25) |
which collapses to the usual intermediate normalization of single reference coupled
cluster theory in case of a one-determinantal reference space.
It is possible to prove size consistency for this ansatz
[15] although
the cluster operator 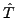 is not connected. In actual applications
[15,16]
the ansatz performed very well.
is not connected. In actual applications
[15,16]
the ansatz performed very well.


Next: An application
Up: Research interest / own
Previous: Cluster Operator Parameterization
Michael Hanrath
2008-08-13